Exercises

1. For each of the following games, each member of the group should find two people who are willing to play the game twenty times. You should give each player index cards labeled with their possible strategies, and you should explain the payoff matrix to each player. For each round of the game, each player is asked to pick a strategy card and put it face down on the table. Then they should turn the cards over and determine the outcome of the game. Your job is to record the strategies chosen and the outcome for each round. At the end of twenty rounds you should compute the total payoff. Bring these records to class on Monday.

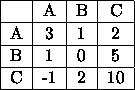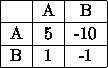
   The three matrix games for each group are attached at the end of the exercises.

2. This is a game which is described in the book Game Theory - A Nontechnical Introduction by Morton Davis.
   1. Two players each put $5 on the table and each flip a coin. Neither player know the outcome of the other players coin flip. Player one goes first. She can either pass or bet an additional $3. If she passes, the players compare their coins. If both are the same, each player takes their $5 back. If not, the player who flipped heads takes the $10. If player one bets the additional $3, player two can either see or fold. If player two folds, player one wins the $10. If player two sees, he adds $3 to the money on the table and then the players compare coins. If they are the same, each player gets their money back. If not, the player who flipped heads takes the $16. Choose two players and play this game 10 times and record the results. Bring the record to class on Monday.
   2. In order to analyze this game as a two-person zero-sum game, we must determine the possible strategies for each player. Each player has four strategies: Player one can always bet, no matter what the coin flip is, player one can always pass no matter what the coin flip is, player one can bet if the coin is heads and pass if it is tails or player one can pass if the coin is heads and bet if it is tails. Thus player one's strategies are :
      • A = always bet
      • B = always pass
      • C = bet for heads, pass for tails
      • D = pass for heads, bet for tails.

      Player two can always see, no matter what the coin flip is, can always fold, no matter what the coin flip is, can see if the coin is heads and fold if it is tails or can fold if the coin is heads and pass if the coin is tails. Thus player two's strategies are:

      • A = always see
      • B = always fold
      • C = see for heads, fold for tails,
      • D = fold for heads, see for tails.

      Now we want to play this game using these four possible strategies for each player as we did in Exercise 1. Each player should then have four cards labelled A through D. Each player should first choose a strategy by placing the appropriate card face down in front of them and then flip the coin. The outcome of the game is then determined by applying the strategies each player has chosen. Play the game ten times this way and record the strategies chosen and the outcome of the game. Bring your record to class on Monday.

Group 1

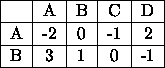
Group 2

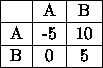
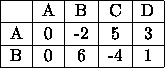
Group 3

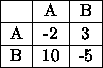
Group 4

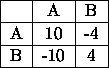
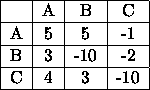
Group 5