Ans. Listen, listen, listen. Be sure to read the CD liner notes as you listen.

Ans.


Ans.

Ans. Bach - nearly any fugue he wrote did this; I Got Rhythm by George Gershwin; Mikrokosmos No. 141 by Bartok; The Thunderer by John Philip Sousa. A horizontal reflection is known as an inversion in music.
Ans. Lean on Me by Bill Withers; The Musical Offering by Johann Sebastian Bach; the ``Hallelujah Chorus'' from the Messiah by George Frideric Handel; Piano Sonata No. 41, ``Minuet and Trio'' in A major by Joseph Haydn. A vertical reflection is known as a retrograde in music.
Ans. Inverses are not in the group. For example, 4^(-1) = 1/4 but 1/4 is not an integer.
Ans. If a musical motif has symmetry H (ph) and symmetry V (pv), then it will have the symmetry obtained by composing these together. But this is H*V=R_180 (p2) so R_180 is guaranteed. Similar arguments work for the other two cases since H*R_180=V and V*R_180=H.
Ans. Both D_4 and S_n are examples of non-commutative groups.
Ans. See the handout from class on Change Ringing .
Ans. (1 2 3), (2 1 3), (2 3 1), (3 2 1), (3 1 2), (1 3 2), (1 2 3). There are only two legitimate extents because at any given stage there are two possible moves: a = (1 2) or b = (2 3). Since a^2 = e and b^2 = e, we must alternate between a and b between changes. This results in either (ab)^3 or (ba)^3 as the only possible extents.
Ans. See the handout from class on Change Ringing . This isn't hard to learn if you recall the moves used to create Plain Bob Minimus, [(ab)^3 ac]^3.
Ans. 6! = 720.
Ans. (1 2 3 4), (2 1 3 4), (2 3 1 4), (2 3 4 1), (2 4 3 1), (2 4 1 3), (2 1 4 3), (1 2 4 3) is the first lead of Double Court Minimus. The second lead is (1 4 2 3), (4 1 2 3), (4 2 1 3), (4 2 3 1), (4 3 2 1), (4 3 1 2), (4 1 3 2), (1 4 3 2) and the third lead is (1 3 4 2), (3 1 4 2), (3 4 1 2), (3 4 2 1), (3 2 4 1), (3 2 1 4), (3 1 2 4), (1 3 2 4), (1 2 3 4). This is a legitimate extent because the first three rules are satisfied. However, rule #4 is not satisfied since Bells 2, 3 and 4 rest in the same position for several changes in a row. Rules 5 and 6 are satisfied.
Ans. 88. See homework #2 (make a table).
Ans. a*b = (2 3 5 1 4), b*a = (4 3 5 2 1), b^3 = b, a^(-1) = (5 1 2 4 3)
Ans. Start looking at higher and higher powers of a. Call the set A = {a, a^2, a^3, a^4, ...}. This set is finite because A is a subset of S_5 which has 5! = 120 elements so eventually we must repeat. Either we keep multiplying until we find a k value where a^k = e, or we will exhaust all 120 elements and a^120 = e.
Ans. a^(-1) = a^3, b^(-1) = b, bab = a implies ab = ba by multiplying by b on the left (or right) of both sides, a^(2011) = a^3 = a^(-1), (ab)^5 = ab (since ab * ab * ab * ab * ab = ab * ba * ab * ba * ab = a^4 * ab = ab).
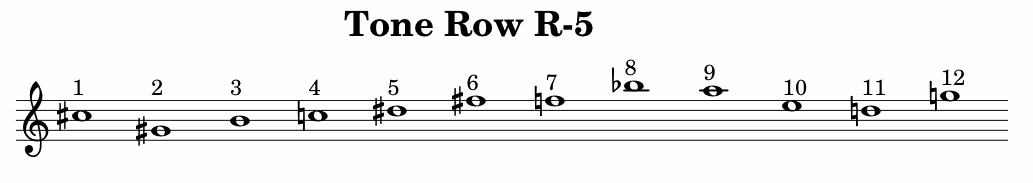
Ans. There are typically 48 different versions of a prime row (including the row itself.) Although this is not the case for every tone row, as some may only generate a total of 24 (e.g., the chromatic scale or two whole tone scales adjoined). The 4 general types of rows (12 of each) are P-n (transposition of P-0 up n half steps), R-n (retrograde of P-n), I-n (inversion of P-n about the starting note or transposition of I-0 up n half steps), RI-n (retrograde inversion of P-n = retrograde of I-n).
Ans. Going up by a minor sixth is going up by 8 half steps, while descending by a major third is 4 half steps. If we think of these numbers as 8 and -4 (minus because we went down), then they are 12 half steps apart, or an octave. But notes that differ by an octave are considered the ''same'' in 12-tone music. If you start on any note and go up by a minor 6th, you will arrive at the same note (in name) as if you had gone down by major 3rd. The intervals m6 and M3 are called inversions of one and other.

Ans. The first and last notes of the row are a tritone apart, just as the primary row used by Schoenberg.




Ans. By symmetry, we may assume that the upper-left corner is 1. By running through the 6 possible cases, one can see that it is never possible to get all of the rows, columns and main diagonals to sum up to 5, the magic constant for n = 2. There is only 1 (up to rotation and reflection) 3 x 3 magic square.
Ans. The magic constant M_8 = 8*(8^2 + 1)/2 = 4*65 = 260.
Ans. Iannis Xenakis in Metastasis.